Evolving the lever rule:
Short-range order configuration modeling of zinc borate glasses
bulletin | Kreidl award abstract
By Brian Topper, Doris Möncke, and Christos Varsamis
Borate and boron-containing glasses have long held a prominent position in glass science since the advent of glass chemistry in the 19th century, when their good glass formability and versatility was discovered.1
Within this glass family, zinc borates were identified early on as a system worth investigating. They were touted as “beautiful, sparkling glasses,” uniquely possessing both high refractive index and good ultraviolet transparency.2 Yet it was only in the past few decades that the short-range order structure of these glasses started to be revealed, mostly thanks to advances in characterization technologies. Understanding the short-range order structure of these glasses is important because the microscopic structural units determine the material’s macroscopic properties.
The earliest structural work on zinc borates came out of Bray’s pioneering lab in the early 1980s.3 Raman spectra reported in the following decade unambiguously showed the presence of boroxol rings, borate rings, metaborate triangles, pyroborate dimers, and orthoborate monomers.4 Contemporary studies confirmed this diverse structural picture of the “zinc metaborate” glass.5,6
In our recent work,7 we aimed to quantify the short-range order (SRO) of the binary zinc borate system across the entire glass-forming range. However, given the results found in References 4–6 and our initial assessment of the vibrational spectra, the system would be extremely difficult to analyze via band decomposition of spectroscopic measurements due to heavy overlap of the spectral features. So, a modified lever rule was used to account for the observation of all types of borate species at a composition lying between the meta- and orthoborate modification levels.
Evolving the lever rule
The conventional lever rule says that when a modifier is introduced into the fully polymerized glass, nonbridging oxygens are created on network formers. As more modifiers are added, the creation of more nonbridging oxygens is necessitated, and so on. The conventional lever rule is depicted in Figure 1a, wherein x denotes the fraction of modifier added to B2O3 and Xi represents the fraction of individual borates units: neutral (N), metaborate (M), pyroborate (P), and orthoborate (O).
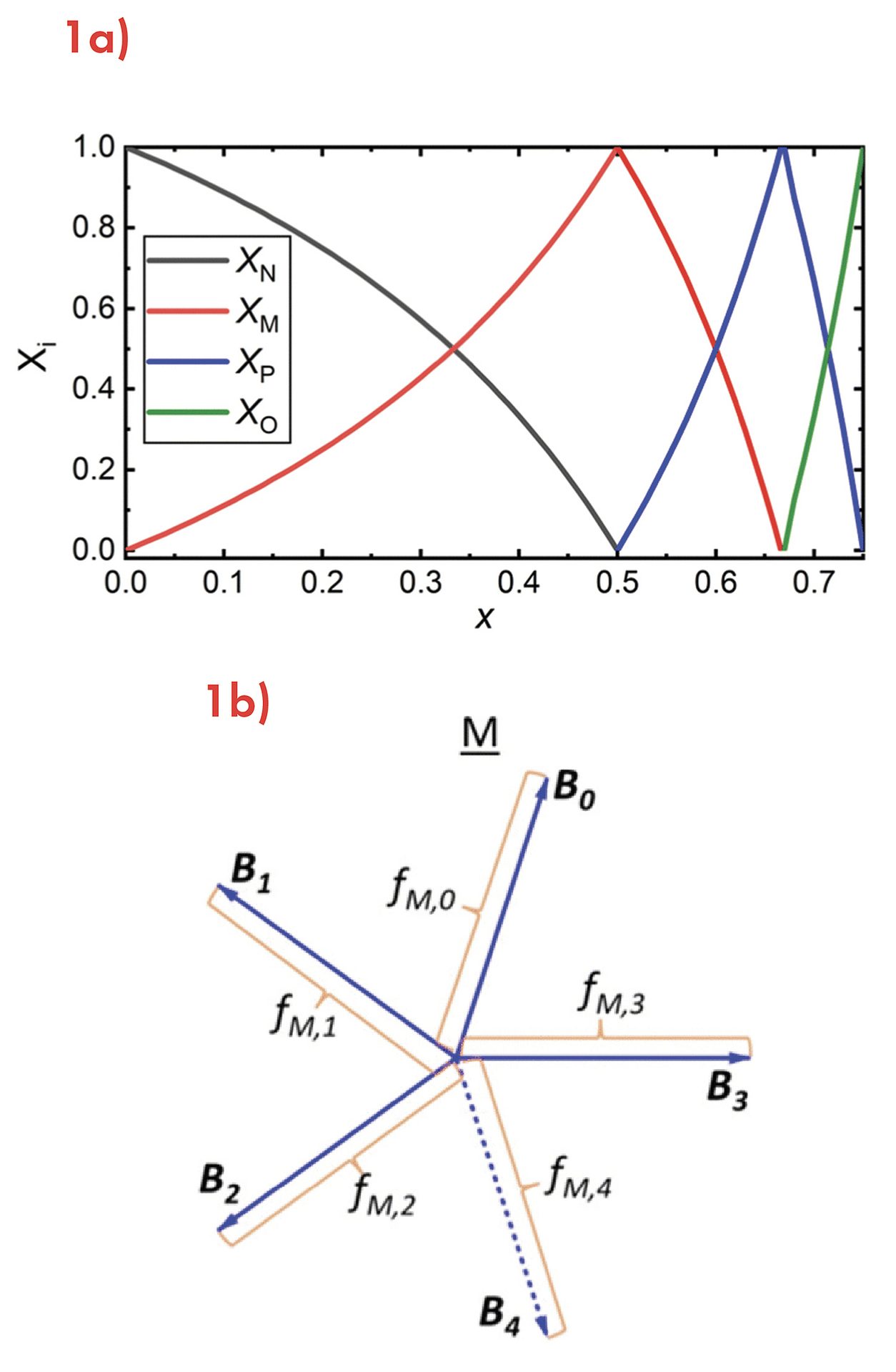
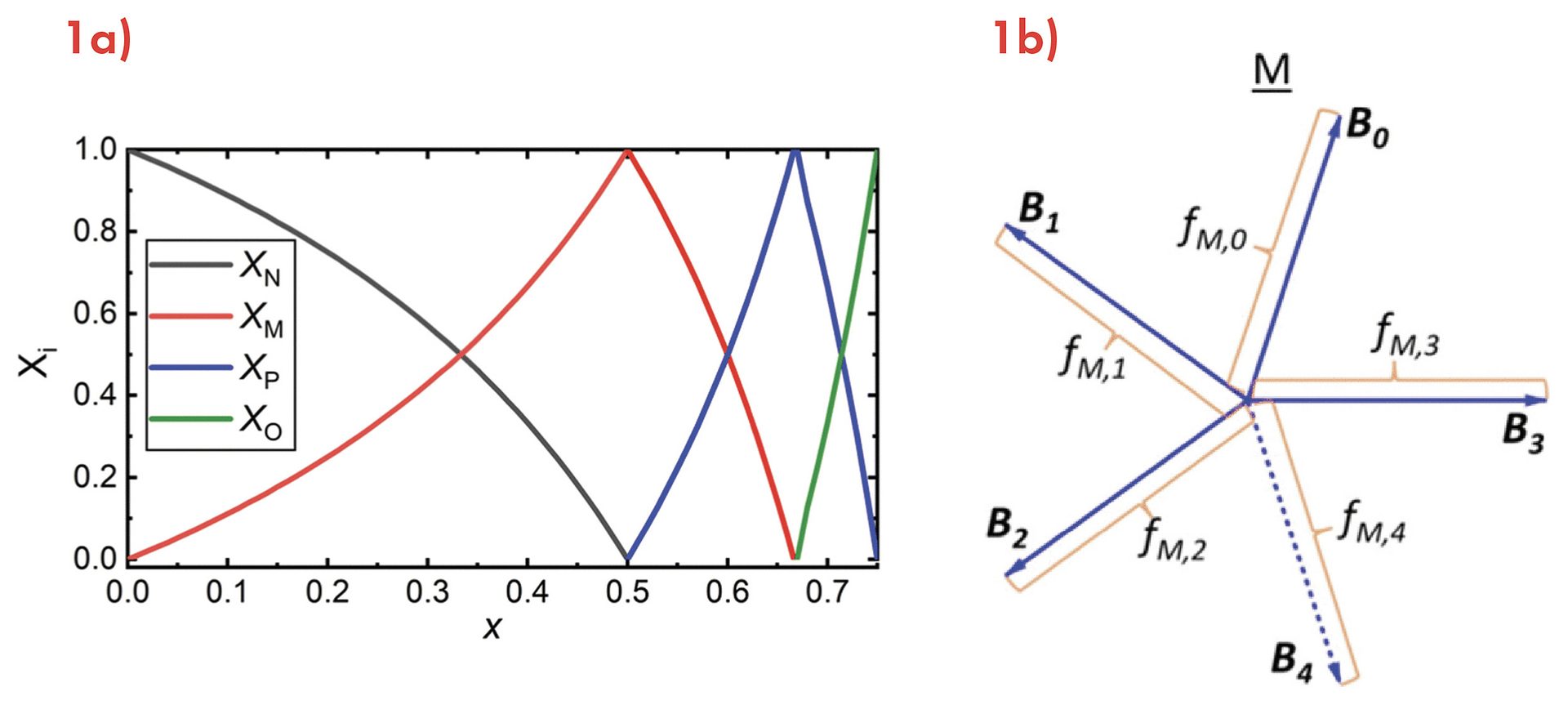
Figure 1. (a) Older notation of the lever rule used in discussion of SRO structure in binary borate glasses xMO–(1-x)B2O3, where the mole fractions Xi(x) (i = N, M, P, O) correspond to the species neutral N (BO1.5)0, metaborate M (BO2)–, pyroborate P (BO2.5)2–, and orthoborate O (BO3)3–.
(b) Theoretical short-range order metaborate configuration with all SRO building block molar fractions (fM,j) of equal length.
Credit: Topper et al., Physical Chemistry Chemical Physics (CC BY-NC 3.0)
The short-range order configuration (SROC) model reimagines each of the nodes as a five-dimensional vector space where the basis is the set of short-range order (SRO) building blocks. The building units are Bj, where j is the number of bridging oxygen atoms per boron (Figure 1b). To apply this model, the molar fraction of the SRO building blocks at each node must be determined experimentally.
Experimental design
We prepared xZnO–(1–x)B2O3 glasses by combining oxide starting materials in platinum crucibles and melting them in an electric furnace. After removal from the furnace, different cooling rates were obtained by either splat quenching or casting and annealing. We then used vibrational spectroscopy (Raman scattering, infrared reflectance), nuclear magnetic resonance, differential scanning calorimetry, and density measurements to characterize the samples. Structurally, the evolution of the borate framework was seen to be sensitive to the ZnO content over the entire glass-forming range.
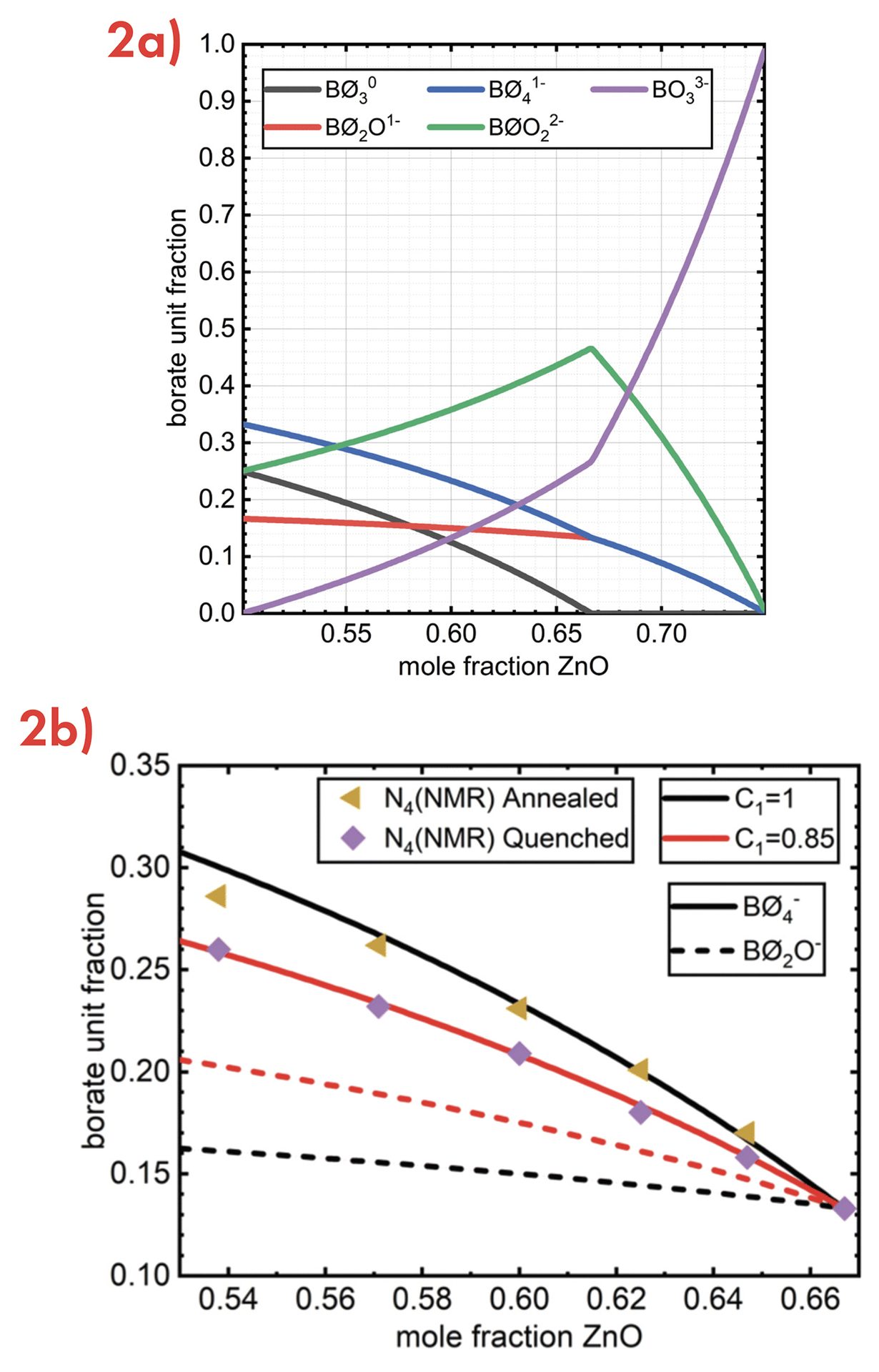
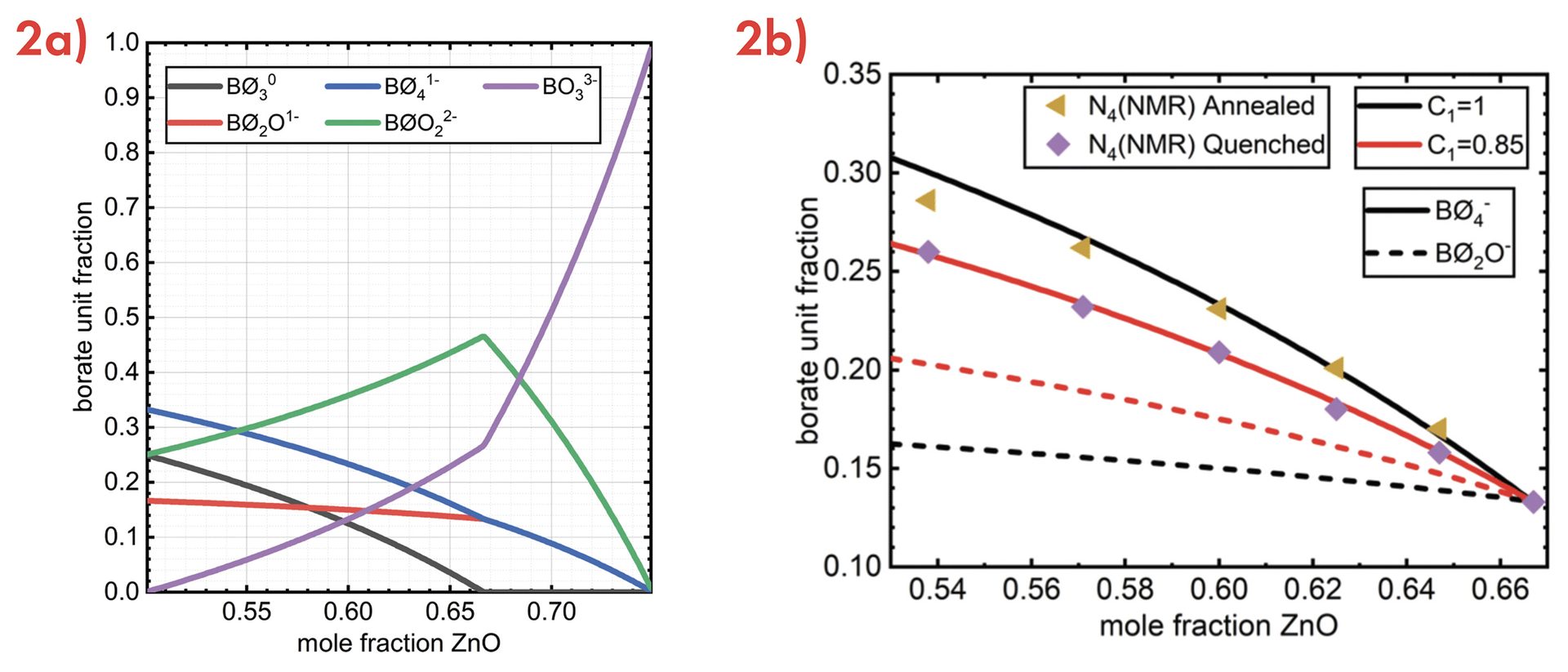
Figure 2. (a) Theoretical fraction of short-range order structural units in binary zinc borate glasses with the fictive temperature-dependent constant set to 1. Note that beyond x=0.667 the fractions of metaborate triangles and tetrahedra are identical.
(b) Comparison of theoretical and experimental (B4 only) fraction of metaborate type species in the bulk glass-forming range; NMR data by R. Youngman, Corning.
Credit: Topper et al., Physical Chemistry Chemical Physics (CC BY-NC 3.0)
Application of the SROC model
The SROC model was carefully applied by using the data from spectroscopic measurements. SROCs for the theoretical M, P, and O species were determined to describe the structure over the interval 0.5 ≤ x ≤ 0.75. By adjusting the unique SROC contribution at a given x by applying the thought process depicted in Figure 1a, the short-range order structure of zinc borate glasses was determined as a function of x over the entire glass-forming range, as depicted in Figure 2a.
Differences between the structure and physical property measurements of annealed and quenched glasses motivated the introduction of a fictive temperature-dependent constant (C1) to capture the cooling rate dependence of the metaborate equilibrium. The fraction of tetrahedral boron as determined by nuclear magnetic resonance is plotted for the quenched (purple diamonds) and annealed (orange triangles) glasses in Figure 2b. The prediction of tetrahedral boron by the model for the bulk glass-forming range by quantifying the structure at x=0.5 and x=0.67 is exceptional, with deviation between modeled and experimental tetrahedral boron being as low as 1%.
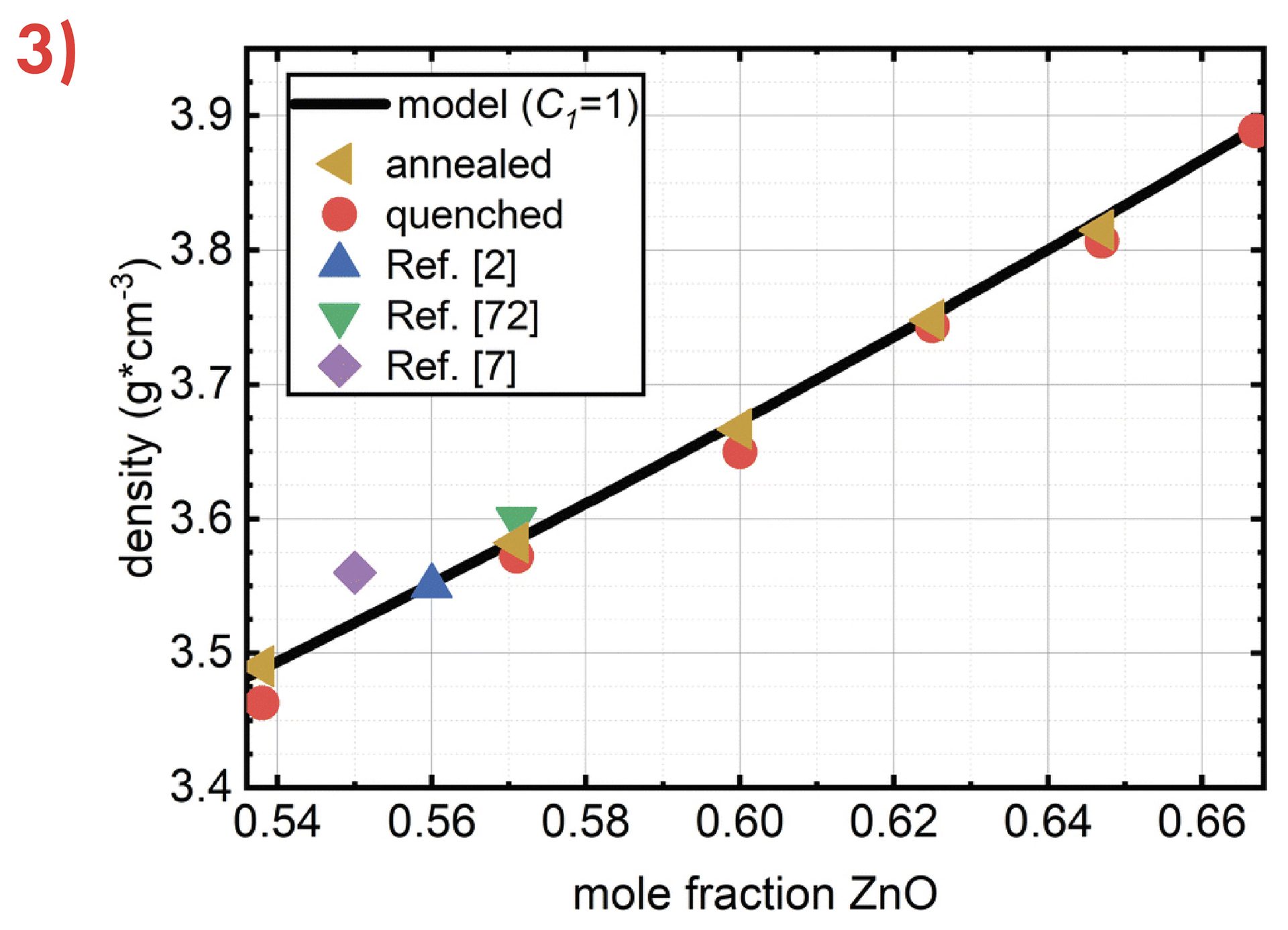
Figure 3. Predicted density using SROCM with fictive temperature dependent constant set to 1 compared to experimental values for binary zinc borate glasses measured in this study (orange triangles and red circles) along with several literature values.
Credit: Topper et al., Physical Chemistry Chemical Physics (CC BY-NC 3.0)
The model’s utility was confirmed by testing its ability to predict glass density (Figure 3). This test was carried out by taking the unique volume fraction and mass of the individual borate units as done by Feller et al.8 As with the model’s prediction of tetrahedral boron, the modeled density mirrored experimental results closely, with the error being approximately 0.004 g•cm–3, or about 0.1%.
Conclusions
The new SROC model developed in this study enabled the determination of binary zinc borate glass structure over the entire composition range. The model is suitable for modified glasses in general, as there is no a priori reason limiting its application to only zinc borates. Ultimately, the model presents itself as a mathematically simple and surprisingly powerful new tool for the glass scientist, particularly with its ability to describe and quantify disproportionation reactions in borate glasses and melts.

F.
Credit:
xx

xx

Fs.
Credit:
xx

F.
Credit:
xx

Fn.
Credit:
xx

F.
Credit:
xx

F.
Credit:
xx
Acknowledgments
We are grateful for Randall Youngman from Corning for 11B NMR measurements.
About the authors:
Brian Topper is a post-doctoral fellow at Clemson University in the group of John Ballato. Doris Möncke, FACerS, is associate professor of glass science and engineering at the New York State College of Ceramics, Alfred University. Christos Varsamis is professor of electrical and electronics engineering at the University of West Attica. Contact Topper.
Editor’s note
Topper presented the 2024 Kreidl Award Lecture at the Glass & Optical Materials Division Annual Meeting on May 21, 2024. Learn more about the conference.
